An oil company blends two input streams of crude oil products alkylate and catalytic cracked to meet demand for weekly contracts for regular (12,000 barrels) mind grade ( 7,500) and premium ( 4,500 barrels) gasoline’s . each week they can purchase up to 15, 000 barrels of alkylate and up to 15,000 barrels of catalytic cracked. Because of demand they can sell all blended gasoline including any production that exceeds its contracted amounts.
Gasoline must meet minimum octane and maximum vapour pressure requirements to be classified as regular mid grade and premium. These rating values are assumed to be the simple weighted average of the crude oil products in the blend and it is assumed there is no waste.
The relevant cost prising octane and vapour pressure data are provided in teh table below:
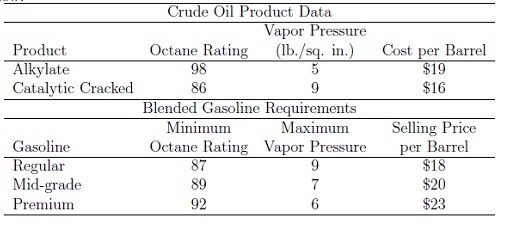
Formulate the linear program the oil company can use to determine how to blend the crude oil products into the commercial gasoline in order to maximize their weekly profit.
Implement your formulation in excel and find the optimal solution nusign solver. Sum marize your solution ( decision variable values and objective function value ) below your for mulation.
For simplicity ( and consistency when I grade) please assume a quarter is 3 month and each month requires 4 weeks.